| Origami Heaven A paperfolding
paradise
The website of
writer and paperfolding designer David Mitchell
x
|
| |
| Nolids and Planar Designs |
| |
| Nolids
are solids of no volume. They can be viewed as composed
of triangular sections of planes which run from the
centre of symmetry of a polyhedron to its edges. In
modular origami nolids paper modules replace and
represent these imaginary planes. Three of these nolids are
special cases in which the triangular planes line up to
form larger sections of planes that are polygonal in
shape. Viewed in this way, the nolid or planar octahedron
is composed of three interpenetrating squares, the nolid
or planar cuboctahedron of four interpenetrating hexagons
and the nolid or planar icosidodecahedron of six
interpenetrating decagons.
Similarly my Four
Octahedra can be seen as being composed of six
interpenetrating 2x1 rectangles and my 12
Interpenetrating Pentagonal Rings as being composed of,
well, the title says it all really!
|
| |
| |
| |
 |
|
Name: Nolid or Planar
Octahedron (2-part) Modules / Paper
shape / Folding geometry: 2 modules from squares
using standard folding geometry.
Designer /
Date: David Mitchell, 1988.
Reference:
542
Diagrams:
On-line diagrams are available on the Modular Designs page of this site.
|
|
| |
 |
|
Name: Nolid
or Planar Octahedron (12-part) - three
interpenetrating squares - not the same modular
method as Robert Neale's similar looking design. Modules / Paper
shape / Folding geometry: 12 modules from silver
rectangles.
Designer /
Date: David Mitchell, 2002
Reference:
247
Diagrams:
Diagrams not yet available.
|
|
| |
 |
|
Name: Nolid
or Planar Tetrahedron Modules / Paper
shape / Folding geometry: 6 modules from silver
rectangles.
Designer /
Date: David Mitchell, 1988 / Ian Harrison.
Reference:
366
Diagrams:
In Mathematical Origami - David Mitchell -
Tarquin publications - ISBN 9781911093169
|
|
| |
 |
|
Name: Nolid
or Planar Cube - robust design developed
from David Brill's more delicate original. Modules / Paper
shape / Folding geometry: 12 modules from silver
rectangles using silver rectangle folding
geometry.
Designer /
Date: David Mitchell, 2012.
Reference:342
Diagrams:
Diagrams not yet available.
|
|
| |
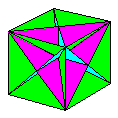 |
|
Name: Nolid
or Planar Cube with Nolid or Planar Tetrahedra Modules / Paper
shape / Folding geometry: Made by adding 30
modules, of two kinds, all folded from silver
rectangles, to David Brill's Nolid Cube.
Designer /
Date: David Mitchell, 2002. Based on a previous
design by Ian Harrison.
Reference:
Diagrams:
In Mathematical Origami - David Mitchell -
Tarquin publications - ISBN 9781911093169
|
|
| |
 |
|
Name: Six
Interpenetrating Planes in a Cube Modules / Paper
shape / Folding geometry: Made by adding 30
modules, of two kinds, all folded from silver
rectangles, to David Brill's Nolid Cube.
Designer /
Date: David Mitchell, 2002.
Reference:
246
Diagrams:
In Mathematical Origami - David Mitchell -
Tarquin publications - ISBN 9781911093169
|
|
| |
 |
|
Name: Nolid
or Planar Cuboctahedron - four
interpenetrating hexagons. Modules / Paper
shape / Folding geometry: 24 modules from
hexagons, squares or double bronze rectangles
using 60/30 degree geometry.
Designer /
Date: David Mitchell, 1988.
Reference:
028
Diagrams:
Mathematical Origami - Tarquin Publications -
ISBN 1-899618-18-X (from double bronze
rectangles) // In Portuguese - Origami
matematicos - Republicao - ISBN 972-570-257-3 // Mathematical Origami - David Mitchell -
Tarquin publications - ISBN 9781911093169
|
|
| |
 |
|
Name: Nolid
or Planar Dodecahedron Modules / Paper
shape / Folding geometry: 30 modules from squares
using standard folding geometry.
Designer /
Date: David Mitchell, 1988.
Reference:
029
Diagrams:
Not yet available.
|
|
| |
 |
|
Name: Nolid
or Planar Icosidodecahedron - six
interpenetrating decagons. Not robust. Modules / Paper
shape / Folding geometry: 60 modules folded from
squares using mock platinum folding geometry.
Designer /
Date: David Mitchell, 1990.
Reference:
065
Diagrams:
In Mathematical Origami - David Mitchell -
Tarquin publications - ISBN 9781911093169
|
|
| |
 |
|
Name: Four
Octahedra - four Robert Neale Octahedra
linked with joining pieces from squares. Larger
versions are possible. . Modules / Paper
shape / Folding geometry: Each Robert Neale
Octahedron is made from 6 modules from squares
using standard folding geometry. Each joining
piece is from a square using standard folding
geometry.
Designer /
Date: David Mitchell, 2016. Update of work from
1995.
Reference:
Diagrams:
Not yet available.
|
|
| |
 |
|
Name: 12
Interpetrating Pentagonal Rings Modules / Paper
shape / Folding geometry: Developed from the
60-part Corner-pocket Sonobe Spiky Star by the
addition of 30 extra modules to link the vertexes
together into rings. All modules from squares
using standard folding geometry.
Designer /
Date: David Mitchell, 1995.
Reference:
Diagrams:
Not yet available.
|
|
| |
| |










