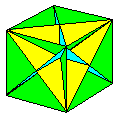
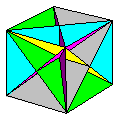
The first edition of
Mathematical Origami was published in 1997 and
had 64 pages. The much more substantial second
edition, published in 2020, has 144. Only six of
the designs in the first edition survive in the
second. The main contents of the second edition
are illustrated below. Except where otherwise
stated all the designs in the book are my own.
Like the first, the second edition
is primarily aimed at mathematics teachers,
although I hope that recreational mathematicians
and modular origami aficionados will also find
much in it to enjoy and enthuse about. The bulk
of the book explains how to fold simple units,
the modules, from ordinary photocopy paper and
how to assemble them, usually without the need
for glue, into polyhedra which are sufficiently
robust to stand up to reasonably rough handling
in a classroom setting.
The advantages of making polyhedra
in this way are:
The process of creating the
necessary starting shapes and of folding
the modules is itself highly mathematical
in nature
Modules / polyhedra can be
made as group projects
All polyhedra built from the
same type of module will have faces to
scale and can therefore be used as the
basis for combination puzzles and to
demonstrate that they will fill (or not
fill) space
Finished polyhedra can be
taken apart and the modules re-used to
build other polyhedra of the same class
The book is divided into three main
sections: Equilateral Deltahedra, Rhombic and
Semi-Rhombic Polyhedra and Planar Polyhedra, with
a few outliers, the Cube, the Regular
Dodecahedron and the Triancontahedron. Of these
the Rhombic and Semi-Rhombic Polyhedra should be
of particular interest as many of them, so far as
I am aware, are not treated of elsewhere.
The modules are folded from a range
of rectangles of differing proportions, squares,
silver rectangles, bronze rectangles, mock
platinum rectanglesn and golden rectangles. Using
a range of rectangles makes folding the modules
much easier than is sometimes the case with other
systems of modular polyhedral design. The book
also shows you how to fold templates to make it
easy to construct rectangles of the correct
proportions in the quantities required.
The second edition of Mathematical
Origami can be purchased in hardcover, paperback
or various ebook formats from: https://www.tarquingroup.com/mathematical-origami-second-edition.html. In case of
difficulty you can contact the sales team via the
web-based contact form at https://www.tarquingroup.com/contacts/
Unfortunately the designer of the
cover randomly decided to include a picture of a
modular paper sculpture (the blue image) which
has nothing whatsoever to do with the contents or
ethos of the book. This design is called Taygeta
and is by Alexander Frolov. The information on
the front and back covers regarding the sections
into which the book is divided is also incorrect.
It relates to an early draft rather than the
finished book.
|



.gif)
.gif)
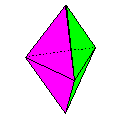
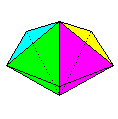
.gif)











.gif)